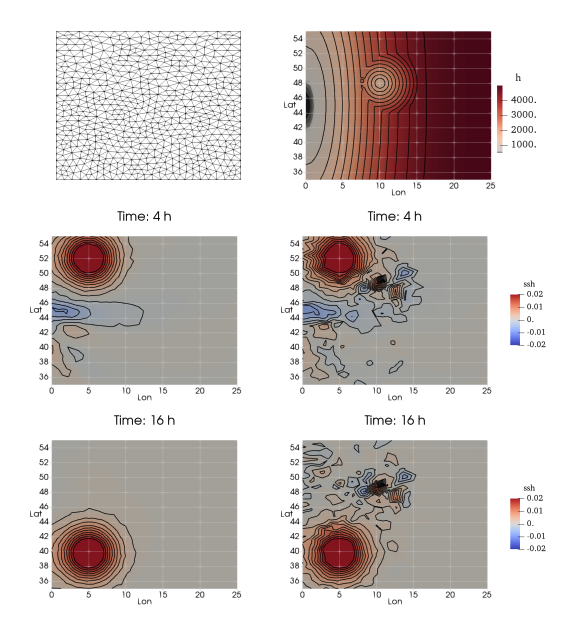
Any numerical approach stems from the necessity of preserving, even at a discrete level, some physical properties of the continuum model. Besides the conservation of typical quantities such as mass, momentum and energy, many works in literature prove that taking care of physical invariants ameliorates the overall quality of the simulations.
Here we concentrate on the preservation of equilibria, entropy and material interfaces.
Well balanced (WB) methods
This family of methods maintains the equilibria solutions of the study system with machine precision over very long and complex simulation times. This allows us to obtain more accurate results with respect to standard schemes, even on coarse meshes, for small perturbations arising around equilibrium profiles.
High order WB schemes for Shallow Water Equations
In the context of preserving stationary states, e.g. lake at rest and moving equilibria, a new formulation of the shallow water system, called Flux Globalization has been introduced. This approach consists in including the integral of the source term in the global flux and reconstructing the new global flux rather than the conservative variables. The resulting scheme is able to preserve a large family of smooth and discontinuous steady state moving equilibria.
Selected result
Transcritical flow: free surface level and discharge compute by means of the GF-WENO5 (red continuous line), WENO5 (black dashed line)
Future work: Multi-dimensional, other hyperbolic equations also containing non-conservative terms
Selected Publications:
WB schemes for Shallow Water Equations written in General Covariant Coordinates
SWE in general covariant coordinates allows us to easily treat various bottom topographies, geometrically described as general embedded manifolds (e.g. spheres or ellipsoids), in reference systems more appropriate than the Cartesian one. We have provided several contributions to the approximation of this problem, including both a fixed reference in General Covariant coordinates and a well balanced DG approximation, and an ALE formulation of the classical Lat-Lon representation approximated with a well-balanced and mass conserving residual distribution approach.
Current work aims at deriving a more general covariant formulation in which the metric terms to be used are provided as input data in the different components of the equation (i.e. the flux, the nonconservative parts and the source term). This way, the physical variables can evolve with respect to the specific definition of the reference system (covariant metric tensor) and, consequently, to the curvature of the considered manifold (Christoffel symbols). Concerning the WB method, balancing involves not only the bathymetry with the water thickness but also metric terms specifying the chosen reference system.
Selected result
Solution at time T = 0.2 of a Riemann problem on a S-shaped river bed. Solutions on the left correnspond to classical shallow water equations in Cartesian coordinates directly computed over the physical S-shaped domain (i.e. the manifold). Solutions on the right refer to shallow water equations in general covariant coordinates over a rectangular computational domain. In particular, the metric employed for these solutions derives from the used transformation map from the rectangular computational domain to the physical S-shaped domain.The solutions obtained with the two approaches are in perfect agreement and two mesh sizes (1.6E-2 and 4.16E-3) are reported to show the grid convergence.
Small amplitude waves interacting with a topographic hump (mountain). Left panel: tidal wave (first column WB DG, second column non-WB DG). Right panel: surge wave (first column WB DG, second column non-WB DG).
Future work: WB Discontinous Galerkin schemes, ALE and covariant formulation, adaptive mesh movement and/or remeshing
Selected Publications:
L. Arpaia, M. Ricchiuto, A.G. Filippini, and R. Pedreros, An efficient 3d/2d-covariant formulation of the spherical shallow water equations: well balanced DG approximation and application to tsunami and storm surge, Ocean Modelling 169, 2021
L. Arpaia and M. Ricchiuto, Well balanced residual distribution for the ALE spherical shallow water equations on moving adaptive meshes, J. Comput. Phys. 405, 2020
M.G. Carlino and E. Gaburro, Well balanced finite volume schemes for shallow water eqations on manifolds (submitted), 2022
WB Finite Volume schemes for astrophysics
In this work we developped a novel second order accurate well balanced (WB) finite volume (FV) scheme for the solution of the general relativistic magnetohydrodynamics (GRMHD) equations and the first order CCZ4 formulation (FO-CCZ4) of the Einstein field equations of general relativity, as well as the fully coupled FO-CCZ4 + GRMHD system. These systems of first order hyperbolic PDEs allow to study the dynamics of the matter and the dynamics of the space-time according to the theory of general relativity.
Numerical results concern the evolution of small perturbations of accretion problems and stable Tolman-Oppenheimer-Volkoff (TOV) neutron stars. Our results show that the well balancing significantly improves the long-time stability of the finite volume scheme compared to a standard one.
Selected result
TOV star simulation with the CCZ4+GRHD fully coupled model with a perturbation of the initial pressure. Left: Hamiltonian and momentum constraints maintained for long computational times with the WB code which instead blow up with the not WB code. Right: oscillation of the density of an inner point of the star due to the initial pressure perturbation.
Future work: 3D fully coupled model, Lagrangian WB schemes
Selected Publications:
E. Gaburro, M.J. Castro, M. Dumbser, A well balanced Finite Volume Scheme for General Relativity, SISC, 2021.
Entropy Preserving schemes
In this work, we aim to develop a fully discrete entropy preserving ADER-Discontinuous Galerkin (ADER-DG) method. To obtain this desired result, we have equipped the space part of the method with entropy correction terms that balance the entropy production in space, inspired by the work of Abgrall. Whereas for the time-discretization we have applied the relaxation approach introduced by Ketcheson that allows modifying the timestep to preserve the entropy to machine precision. Up to our knowledge, it is the first time that a provable fully discrete entropy preserving ADER-DG scheme is constructed.
Future work: Coupling our entropy preserving ADER-DG scheme with a provably entropy dissipative and robust limiter technique, devising a strategy to enforce locally the fully discrete preservation (space AND time)
Selected Publications:
E. Gaburro, P. Öffner, M. Ricchiuto, D. Torlo, High order entropy preserving ADER-DG schemes, submitted, 2022.
Galilean invariance and interface tracking with Direct ALE schemes
The use of Lagrangian schemes allows the writing of numerical schemes that are Galilean invariant and suitable for interface tracking.
We are developing a novel family of Direct ALE schemes with high order of accuracy over moving Voronoi meshes with topology changes. Thanks to the high order of accuracy and to the optimal quality of the moving meshes the new family of schemes offers high resolutions over long simulation times.
Future work: Many works are in preparation on this topic to increase the robustness and the efficiency of the scheme (new mesh optimization technqiues, new limiter both for FV and DG, parallelization, well balancing, inclusion of more complex equations)
Selected Publications:
E. Gaburro, A unified framework for the solution of hyperbolic PDE systems using high order direct Arbitrary-Lagrangian-Eulerian schemes on moving unstructured meshes with topology change, ACME 2020.