Character Varieties

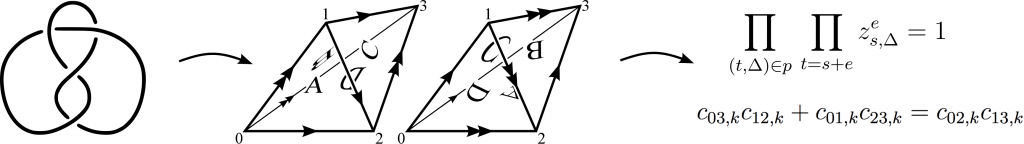
Our initial motivation was to unify the theory of spherical CR-structures and the theory of hyperbolic structures on 3-manifolds, by viewing them in the common framework of so-called flag structures. In terms of holonomy representations, which take values in \(\mathrm{SL}(2,\mathbb{C})\) and \(SU(2,1)\) for hyperbolic and spherical CR-structures respectively, we view these two groups as subgroups of \(SL(3,\mathbb{C})\).

We intend to study representations of 3-manifold groups into \(SL(3,\mathbb{C})\) that come from geometric structures on 3-manifolds. More specifically, we study representations that come from a triangulation of the 3-manifold. With a suitable interpretation, one can think of both hyperbolic and spherical CR structures as being described by associating to each vertex of the tetrahedra in the triangulation a flag, i.e. a point in the complex projective plane together with a projective line that contains that point. We shall refer to such a structure as a flag-structure on the 3- manifold. These are parametrized by the invariants of quadruples of flags. The gluing of tetrahedra in the triangulation imposes certain relations between the invariants, which can be expressed as a system of algebraic equations.

One goal is to develop efficient methods to solve these compatibility equations, and to develop a computer program that finds all solutions and computes their invariants. Eventually, we intend to study the existence and rigidity properties of the solutions, and to give a geometric interpretation of the results.

slider1-1024x144-1024x144

Related publications

  • [PDF] A. Guilloux, “Volume of representations and birationality of peripheral holonomy,” Experimental Mathematics, 2017.
    [Bibtex] 
    @article{guilloux:hal-01370287,
TITLE = {{Volume of representations and birationality of peripheral holonomy}},
AUTHOR = {Guilloux, Antonin},
URL = {https://hal.archives-ouvertes.fr/hal-01370287},
JOURNAL = {{Experimental Mathematics}},
PUBLISHER = {{Taylor \& Francis}},
YEAR = {2017},
MONTH = May,
PDF = {https://hal.archives-ouvertes.fr/hal-01370287/file/VolBirra.pdf},
HAL_ID = {hal-01370287},
HAL_VERSION = {v1},
}
  • [DOI] E. Falbel, A. Guilloux, P. Koseleff, F. Rouillier, and M. Thistlethwaite, “Character Varieties For SL(3,C): The Figure Eight Knot,” Experimental Mathematics, vol. 25, iss. 2, p. 17, 2016.
    [Bibtex] 
    @article{falbel:hal-01362208,
TITLE = {{Character Varieties For SL(3,C): The Figure Eight Knot}},
AUTHOR = {Falbel, Elisha and Guilloux, Antonin and Koseleff, Pierre-Vincent and Rouillier, Fabrice and Thistlethwaite, Morwen},
URL = {https://hal.inria.fr/hal-01362208},
JOURNAL = {{Experimental Mathematics}},
PUBLISHER = {{Taylor \& Francis}},
VOLUME = {25},
NUMBER = {2},
PAGES = {17},
YEAR = {2016},
DOI = {10.1080/10586458.2015.1068249},
KEYWORDS = { figure eight knot ; character varieties ; decorated representations ; },
HAL_ID = {hal-01362208},
HAL_VERSION = {v1}
}
  • [DOI] E. Falbel and J. M. Veloso, “Diffeomorphisms preserving R-circles in three dimensional CR manifolds,” Mathematical News / Mathematische Nachrichten, vol. 289, iss. 8-9, pp. 962-973, 2016.
    [Bibtex] 
    @article{falbel:hal-01374792,
TITLE = {{Diffeomorphisms preserving R-circles in three dimensional CR manifolds}},
AUTHOR = {Falbel, Elisha and Veloso, Jose M.},
URL = {https://hal.archives-ouvertes.fr/hal-01374792},
JOURNAL = {{Mathematical News / Mathematische Nachrichten}},
PUBLISHER = {{Wiley-VCH Verlag}},
VOLUME = {289},
NUMBER = {8-9},
PAGES = {962-973},
YEAR = {2016},
DOI = {10.1002/mana.201400301},
HAL_ID = {hal-01374792},
HAL_VERSION = {v1},
}
  • E. Falbel and Q. Wang, “Duality and invariants of representations of fundamental groups of 3-manifolds into PGL(3,C),” Bulletin of the London Mathematical Society, 2016.
    [Bibtex] 
    @article{falbel:hal-01374795,
TITLE = {{Duality and invariants of representations of fundamental groups of 3-manifolds into PGL(3,C)}},
AUTHOR = {Falbel, Elisha and Wang, Qingxue},
URL = {https://hal.archives-ouvertes.fr/hal-01374795},
JOURNAL = {{Bulletin of the London Mathematical Society}},
PUBLISHER = {{London Mathematical Society}},
YEAR = {2016},
HAL_ID = {hal-01374795},
HAL_VERSION = {v1},
}
  • [DOI] E. Falbel, P. Koseleff, and F. Rouillier, “Representations of fundamental groups of 3-manifolds into PGL(3,C): Exact computations in low complexity,” Geometriae Dedicata, vol. 177, iss. 1, p. 52, 2015.
    [Bibtex] 
    @article{falbel:hal-00908843,
TITLE = {{Representations of fundamental groups of 3-manifolds into PGL(3,C): Exact computations in low complexity}},
AUTHOR = {Falbel, Elisha and Koseleff, Pierre-Vincent and Rouillier, Fabrice},
URL = {https://hal.inria.fr/hal-00908843},
JOURNAL = {{Geometriae Dedicata}},
PUBLISHER = {{Springer Verlag}},
VOLUME = {177},
NUMBER = {1},
PAGES = {52},
YEAR = {2015},
MONTH = Aug,
DOI = {10.1007/s10711-014-9987-x},
HAL_ID = {hal-00908843},
HAL_VERSION = {v1},
}
  • [PDF] [DOI] M. Deraux and E. Falbel, “Complex hyperbolic geometry of the figure eight knot,” Geometry and Topology, vol. 19, p. 237–293, 2015.
    [Bibtex] 
    @article{deraux:hal-00805427,
TITLE = {{Complex hyperbolic geometry of the figure eight knot}},
AUTHOR = {Deraux, Martin and Falbel, Elisha},
URL = {https://hal.archives-ouvertes.fr/hal-00805427},
JOURNAL = {{Geometry and Topology}},
HAL_LOCAL_REFERENCE = {IF\_PREPUB},
PUBLISHER = {{Mathematical Sciences Publishers}},
VOLUME = {19},
PAGES = {237--293},
YEAR = {2015},
MONTH = Feb,
DOI = {10.2140/gt.2015.19.237},
PDF = {https://hal.archives-ouvertes.fr/hal-00805427/file/figure8.pdf},
HAL_ID = {hal-00805427},
HAL_VERSION = {v2},
}
  • [PDF] E. Falbel and R. Santos Thebaldi, “A Flag structure on a cusped hyperbolic 3-manifold with unipotent holonomy.,” Pacific Journal of Mathematics, vol. 278, iss. 1, pp. 51-78, 2015.
    [Bibtex] 
    @article{falbel:hal-00958255,
TITLE = {{A Flag structure on a cusped hyperbolic 3-manifold with unipotent holonomy.}},
AUTHOR = {Falbel, Elisha and Santos Thebaldi, Rafael},
URL = {https://hal.archives-ouvertes.fr/hal-00958255},
JOURNAL = {{Pacific Journal of Mathematics}},
PUBLISHER = {{Mathematical Sciences Publishers}},
VOLUME = {278},
NUMBER = {1},
PAGES = {51-78},
YEAR = {2015},
KEYWORDS = {SL(3 ; cusped 3-manifold ; Geometric structure ; Flag structure ; R)},
PDF = {https://hal.archives-ouvertes.fr/hal-00958255/file/tetraprojective.pdf},
HAL_ID = {hal-00958255},
HAL_VERSION = {v1},
}
  • [PDF] A. Guilloux, “Représentations et Structures Géométriques,” Accreditation to supervise research PhD Thesis, 2015.
    [Bibtex] 
    @phdthesis{guilloux:tel-01370296,
TITLE = {{Repr{\'e}sentations et Structures G{\'e}om{\'e}triques}},
AUTHOR = {Guilloux, Antonin},
URL = {https://hal.archives-ouvertes.fr/tel-01370296},
SCHOOL = {{UPMC - Universit{\'e} Paris 6 Pierre et Marie Curie}},
YEAR = {2015},
MONTH = Nov,
KEYWORDS = {Character variety ; 3-manifolds ; Geometric structures ; structures g{\'e}om{\'e}triques ; repr{\'e}sentation ; Vari{\'e}t{\'e} de caract{\`e}res},
TYPE = {Accreditation to supervise research},
PDF = {https://hal.archives-ouvertes.fr/tel-01370296/file/hdr.pdf},
HAL_ID = {tel-01370296},
HAL_VERSION = {v1},
}
  • E. Falbel and Q. Wang, “A combinatorial invariant for spherical cr structures.,” Asian j. math., vol. 17, iss. 2, p. 391–422, 2012.
    [Bibtex] 
    @article{FW,
Author = {E. Falbel and Q. Wang },
Date-Added = {2015-01-13 13:49:42 +0100},
Date-Modified = {2015-01-13 13:50:47 +0100},
Journal = {Asian J. Math.},
Number = {2},
Pages = {391--422},
Title = {A combinatorial invariant for spherical CR structures.},
Volume = {17},
Year = {2012}
}
  • E. Falbel and J. M. Veloso, “A lorentz form associated to contact sub-conformal and cr manifolds.,” Kodai math. j., vol. 37, iss. 2, p. 405–426, 2014.
    [Bibtex] 
    @article{FV,
Author = {E. Falbel and J. M. Veloso},
Date-Added = {2015-01-13 13:48:03 +0100},
Date-Modified = {2015-01-13 13:49:25 +0100},
Journal = {Kodai Math. J.},
Number = {2},
Pages = {405--426},
Title = {A Lorentz form associated to contact sub-conformal and CR manifolds.},
Volume = {37},
Year = {2014}}
  • E. Falbel and J. Wang, “Branched spherical cr structures on the complement of the figure-eight knot,” Michigan math. j., vol. 63, iss. 3, p. 635–667, 2014.
    [Bibtex] 
    @article{FWbranch,
Author = {Falbel, E. and J. Wang},
Date-Added = {2015-01-13 13:41:23 +0100},
Date-Modified = {2015-01-13 13:47:45 +0100},
Journal = {Michigan Math. J.},
Number = {3},
Pages = { 635--667},
Publisher = { },
Title = {Branched spherical CR structures on the complement of the figure-eight knot},
Volume = {63},
Year = {2014}}
  • N. Bergeron, E. Falbel, and A. Guilloux, “Tetrahedra of flags, volume and homology of SL(3),” Geometry and topology, vol. 18, 2014.
    [Bibtex] 
    @article{BFG14,
Author = {Bergeron, N. and Falbel, E. and Guilloux, A.},
Journal = {Geometry and Topology},
Keywords = {Mathematics - Geometric Topology, Mathematics - K-Theory and Homology},
Pages = 1911-1971,
Title = {{Tetrahedra of flags, volume and homology of SL(3)}},
Volume = {18},
Year = 2014}
  • A. Guilloux, “Deformation of hyperbolic manifolds in and discreteness of the peripheral representations,” Proceedings of the american mathematical society, 2014.
    [Bibtex] 
    @article{Gui14,
Author = {Guilloux, A.},
Journal = {Proceedings of the American Mathematical Society},
Title = {{Deformation of hyperbolic manifolds in and discreteness of the peripheral representations}},
Year = 2014}
  • [DOI] N. Bergeron, A. Guilloux, E. Falbel, P. Koseleff, and F. Rouillier, “Local rigidity for sl (3,c) representations of 3-manifolds groups,” Experimental Mathematics, vol. 22, iss. 4, p. 10, 2013.
    [Bibtex] 
    @article{BGFKR13,
Author = {Bergeron, Nicolas and Guilloux, Antonin and Falbel, Elisha and Koseleff, Pierre-Vincent and Rouillier, Fabrice},
Doi = {10.1080/10586458.2013.832441},
Hal_Id = {hal-00803837},
Hal_Version = {v1},
Journal = {{Experimental Mathematics}},
Number = {4},
Pages = {10},
Publisher = {{AK Peters}},
Title = {Local rigidity for SL (3,C) representations of 3-manifolds groups},
Url = {https://hal.inria.fr/hal-00803837},
Volume = {22},
Year = {2013},
Bdsk-Url-1 = {https://hal.inria.fr/hal-00803837},
Bdsk-Url-2 = {http://dx.doi.org/10.1080/10586458.2013.832441}}

 

Comments are closed.