Algebraic computations have tremendously been used in Robotics, especially in kinematics, since the last quarter of the 20th century. For example, one can find algebraic proofs for the 40 possible solutions to the direct kinematics problem for steward platforms and companion experiments based on Gröbner basis computations. On the one hand, hard general kinematics problems involve too many variables for pure algebraic methods to be used in place of existing numerical or semi-numerical methods everywhere and everytime, and on the other hand, global algebraic studies allow to propose exhaustive classifications that cannot be reached by other methods,for some quite large classes.
Robotics is a long-standing collaborative work with LS2N (Laboratory of Numerical Sciences of Nantes). Work has recently focused on the offline study of mechanisms, mostly parallel, their singularities or at least some types of singularities (cuspidals robots).
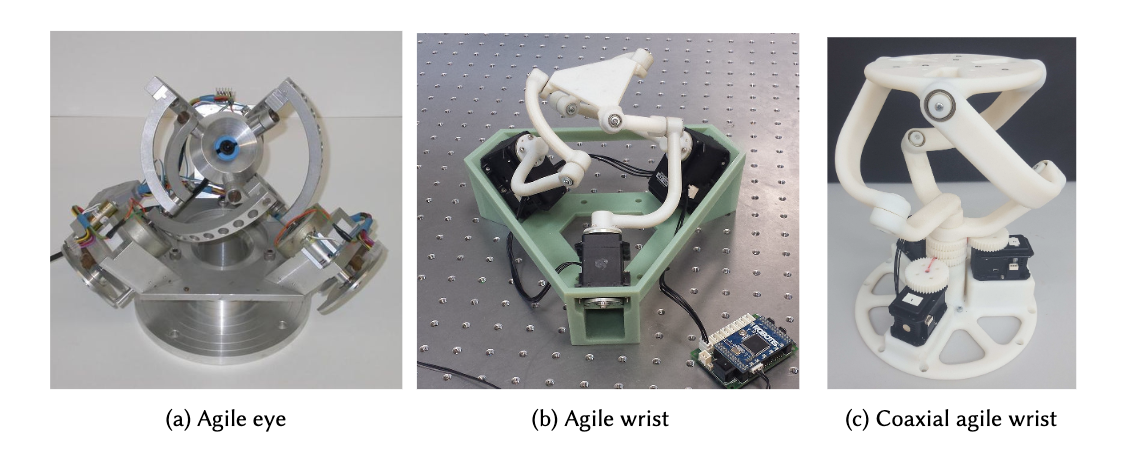
For most parallel or serial manipulators, pose variables and joints variables are linked by algebraic equations and thus lie an algebraic variety. The two-kinematics problems (the direct kinematics problem – DKP- and the inverse kinematics problem – IKP) consist in studying the preimage of the projection of this algebraic variety onto a subset of unknowns. Solving the DKP remains to computing the possible positions for a given set of joint variables values while solving the IKP remains to computing the possible joints variables values for a given position. Algebraic methods have been deeply used in several situations for studying parallel and serial mechanisms, but finally their use stays quite confidential in the design process. Cylindrical Algebraic Decomposition coupled with variable’s eliminations by means of Gröbner based computations can be used to model the workspace, the joint space and the computation of singularities. On the one hand, such methods suffer immediately when increasing the number of parameters or when working with imprecise data. On the other hand, when the problem can be handled, they might provide full and exhaustive classifications. The tools we use in that context depend mainly on the resolution of parameter-based systems and therefore of study-dependent curves or flat algebraic surfaces (2 or 3 parameters), thus joining our thematic Computational Geometry.
Since 2020, our research takes place in an industrial project driven by Safran Défense and Electronics and involving the REV team at LS2N (CNRS – Nantes – France) to design parallel manipulators for some stabilization problems. For obvious reasons, only few information/publications are publicly available.
Related publications :
-
![[DOI]](https://team.inria.fr/ouragan/wp-content/plugins/papercite/img/external.png) A. Lê, F. Rouillier, D. Chablat, and G. Rance, “On the Certification of the Kinematics of 3-DOF Spherical Parallel Manipulators,” Maple Transactions, vol. 3, iss. 2, 2023.
A. Lê, F. Rouillier, D. Chablat, and G. Rance, “On the Certification of the Kinematics of 3-DOF Spherical Parallel Manipulators,” Maple Transactions, vol. 3, iss. 2, 2023.
[Bibtex]@article{le:hal-04189637, TITLE = {{On the Certification of the Kinematics of 3-DOF Spherical Parallel Manipulators}}, AUTHOR = {L{\^e}, Alexandre and Rouillier, Fabrice and Chablat, Damien and Rance, Guillaume}, URL = {https://hal.science/hal-04189637}, JOURNAL = {{Maple Transactions}}, VOLUME = {3}, NUMBER = {2}, YEAR = {2023}, MONTH = Aug, DOI = {10.5206/mt.v3i2.15660}, HAL_ID = {hal-04189637}, HAL_VERSION = {v1}, }
-
![[PDF]](https://team.inria.fr/ouragan/wp-content/plugins/papercite/img/pdf.png)
![[DOI]](https://team.inria.fr/ouragan/wp-content/plugins/papercite/img/external.png) D. Chablat, G. Moroz, F. Rouillier, and P. Wenger, “Using Maple to analyse parallel robots,” in Maple in Mathematics Education and Research, J. Gerhard and I. Kotsireas, Eds., Springer, Cham, 2020, pp. 50-64.
D. Chablat, G. Moroz, F. Rouillier, and P. Wenger, “Using Maple to analyse parallel robots,” in Maple in Mathematics Education and Research, J. Gerhard and I. Kotsireas, Eds., Springer, Cham, 2020, pp. 50-64.
[Bibtex]@incollection{chablat:hal-02289699, TITLE = {{Using Maple to analyse parallel robots}}, AUTHOR = {Chablat, Damien and Moroz, Guillaume and Rouillier, Fabrice and Wenger, Philippe}, URL = {https://hal.archives-ouvertes.fr/hal-02289699}, BOOKTITLE = {{Maple in Mathematics Education and Research}}, EDITOR = {J{\"u}rgen Gerhard and Ilias Kotsireas}, PUBLISHER = {{Springer, Cham}}, SERIES = {Maple in Mathematics Education and Research}, PAGES = {50-64}, YEAR = {2020}, MONTH = Feb, DOI = {10.1007/978-3-030-41258-6\_4}, PDF = {https://hal.archives-ouvertes.fr/hal-02289699/file/2019_Maple_Conference.pdf}, HAL_ID = {hal-02289699}, HAL_VERSION = {v1}, }
-
![[PDF]](https://team.inria.fr/ouragan/wp-content/plugins/papercite/img/pdf.png)
![[DOI]](https://team.inria.fr/ouragan/wp-content/plugins/papercite/img/external.png) R. Jha, D. Chablat, L. Baron, F. Rouillier, and G. Moroz, “Workspace, Joint space and Singularities of a family of Delta-Like Robot,” Mechanism and Machine Theory, vol. 127, pp. 73-95, 2018.
R. Jha, D. Chablat, L. Baron, F. Rouillier, and G. Moroz, “Workspace, Joint space and Singularities of a family of Delta-Like Robot,” Mechanism and Machine Theory, vol. 127, pp. 73-95, 2018.
[Bibtex]@article{jha:hal-01796066, TITLE = {{Workspace, Joint space and Singularities of a family of Delta-Like Robot}}, AUTHOR = {Jha, Ranjan and Chablat, Damien and Baron, Luc and Rouillier, Fabrice and Moroz, Guillaume}, URL = {https://hal.archives-ouvertes.fr/hal-01796066}, JOURNAL = {{Mechanism and Machine Theory}}, PUBLISHER = {{Elsevier}}, VOLUME = {127}, PAGES = {73-95}, YEAR = {2018}, MONTH = Sep, DOI = {10.1016/j.mechmachtheory.2018.05.004}, KEYWORDS = {Parallel robot ; Groebner basis ; Cylindrical algebraic decomposition ; Delta-like robot ; Workspace ; Singularities}, PDF = {https://hal.archives-ouvertes.fr/hal-01796066/file/MMT_Delta_Like_Robot.pdf}, HAL_ID = {hal-01796066}, HAL_VERSION = {v1}, }