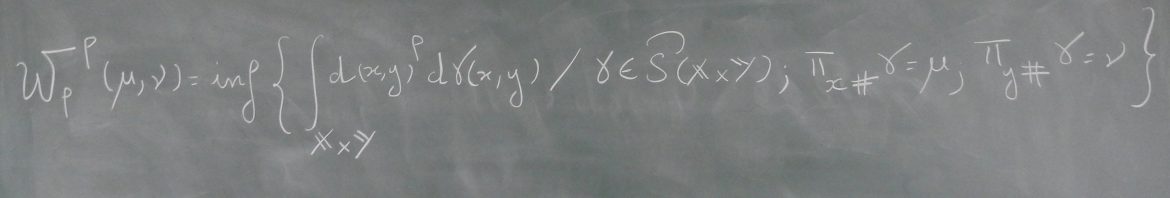Team seminars are called MokaMeetings. They are organized on a monthly basis. Subscribe to the mailing list to be notified about upcoming seminars.
The list of past seminars is available here.
- Mokameeting du 20 mars 2024 : Laurent Pfeiffer (INRIA Saclay) et Anna Korba (ENSAE)
Mercredi 20 mars, nous aurons le plaisir d’écouter Laurent Pfeiffer de l’INRIA Saclay ainsi qu’Anna Korba de l’ENSAE.
La séance aura lieu à 14h dans la salle Jacques-Louis Lions 2 à l’Inria Paris.Laurent Pfeiffer (Inria Saclay)
On the Frank-Wolfe algorithm and its application to non-atomic potential games
Résumé: I will review two recent results concerning the Frank-Wolfe algorithm, an algorithm dating from the 1950 among of the very first methods for solving constrained optimization problems: following .K. Liu, Pfeiffer. Mean field optimization problems: stability results and Lagrangian discretization. Preprint, 2023.
Anna Korba (ENSAE/CREST)
Sampling through optimization of discrepancies
Résumé: Sampling from a target measure when only partial information is available (e.g. unnormalized density as in Bayesian inference, or true samples as in generative modeling ) is a fundamental problem in computational statistics and machine learning. The sampling problem can be formulated as an optimization over the space of probability distributions of a well-chosen discrepancy (e.g. a divergence or distance).
In this talk, we’ll discuss several properties of sampling algorithms for some choices of discrepancies (well-known ones, or novel proxies), both regarding their optimization and quantization aspects.