Today the main goal of GAMMA project is to certify the numerical results of the Numerical Simulation Pipeline and to provide high fidelity numerical solutions. To do so, the team focuses on state-of-the-art meshing technologies and advanced numerical methods. High-order mesh and solution enable High-Fidelity of the Numerical Simulation Pipeline. The certification of the Numerical Simulation Pipeline is based on uncertainty quantification, mesh adaptation to optimally control discretization errors and mesh converged numerical solutions. To this end, numerous research topics are defined regarding:
- mesh generation algorithms (generic, adaptive and high-order),
- numerical schemes and flow solvers,
- error estimates,
- parallel computing,
- visualisation.
Research themes
- Geometric Modeling:
- High-fidelity discrete CAD kernel.
- Continuous parametric CAD kernel.
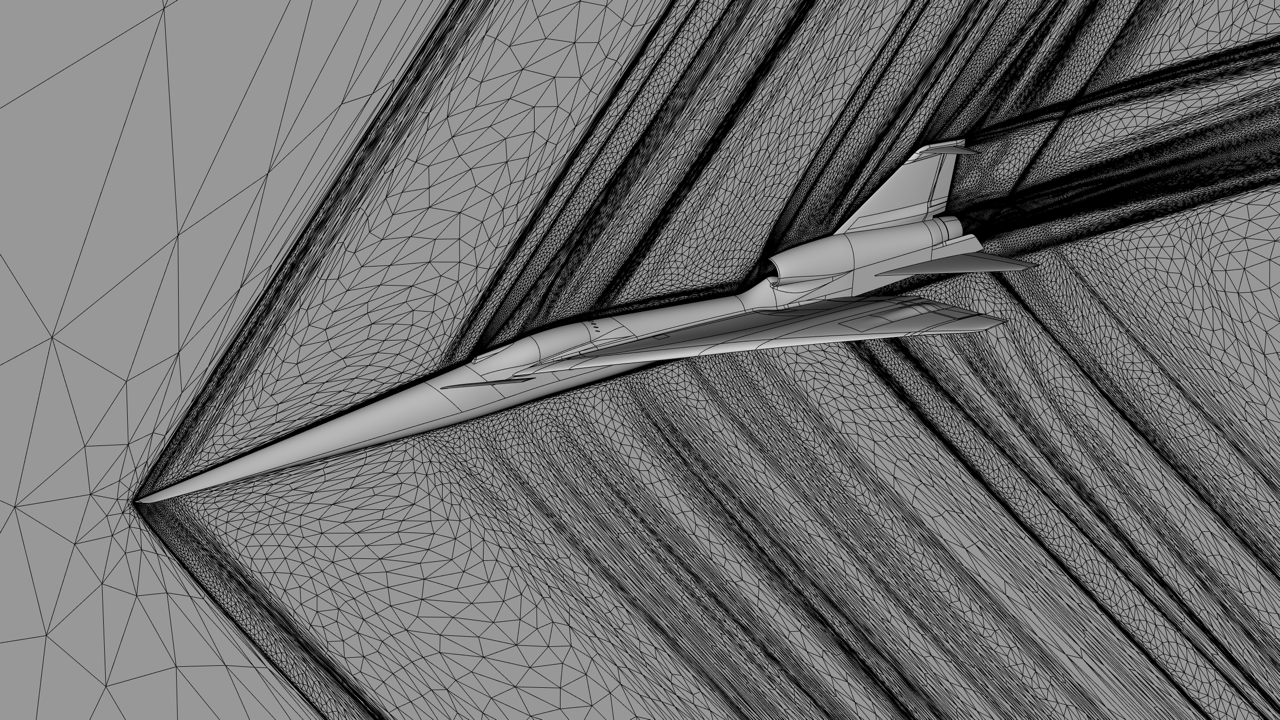
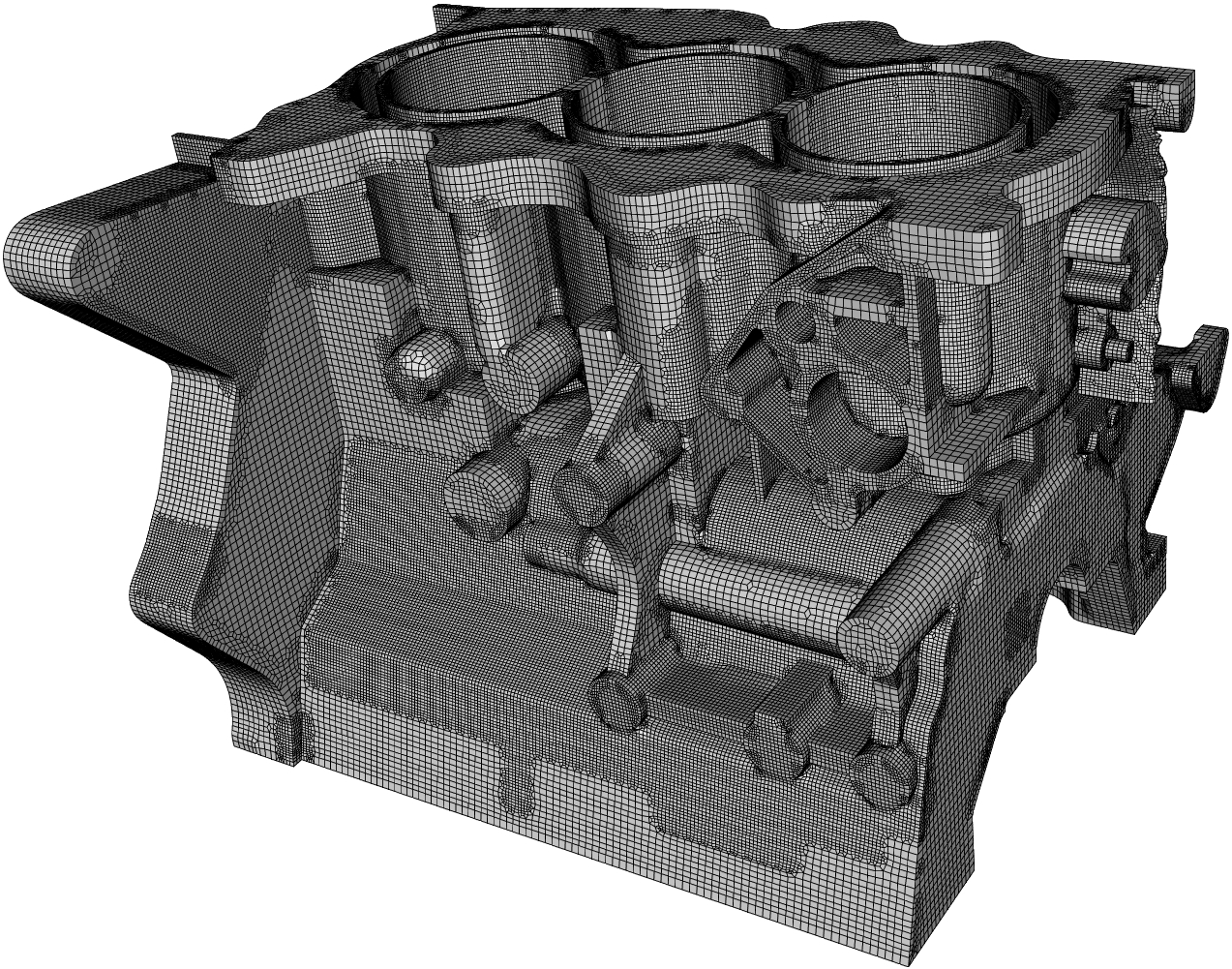
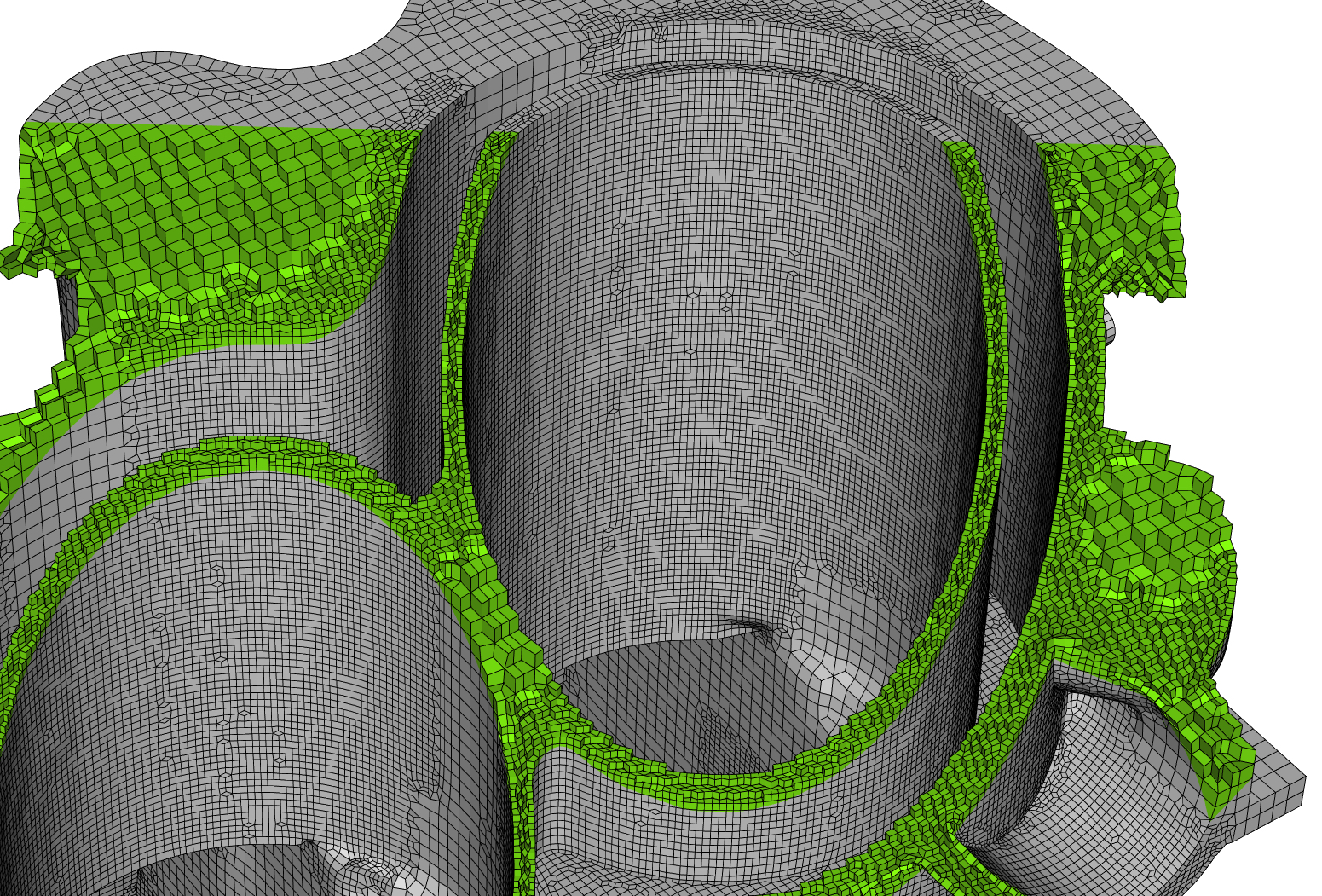
- Enhanced Generic Meshing Algorithm:
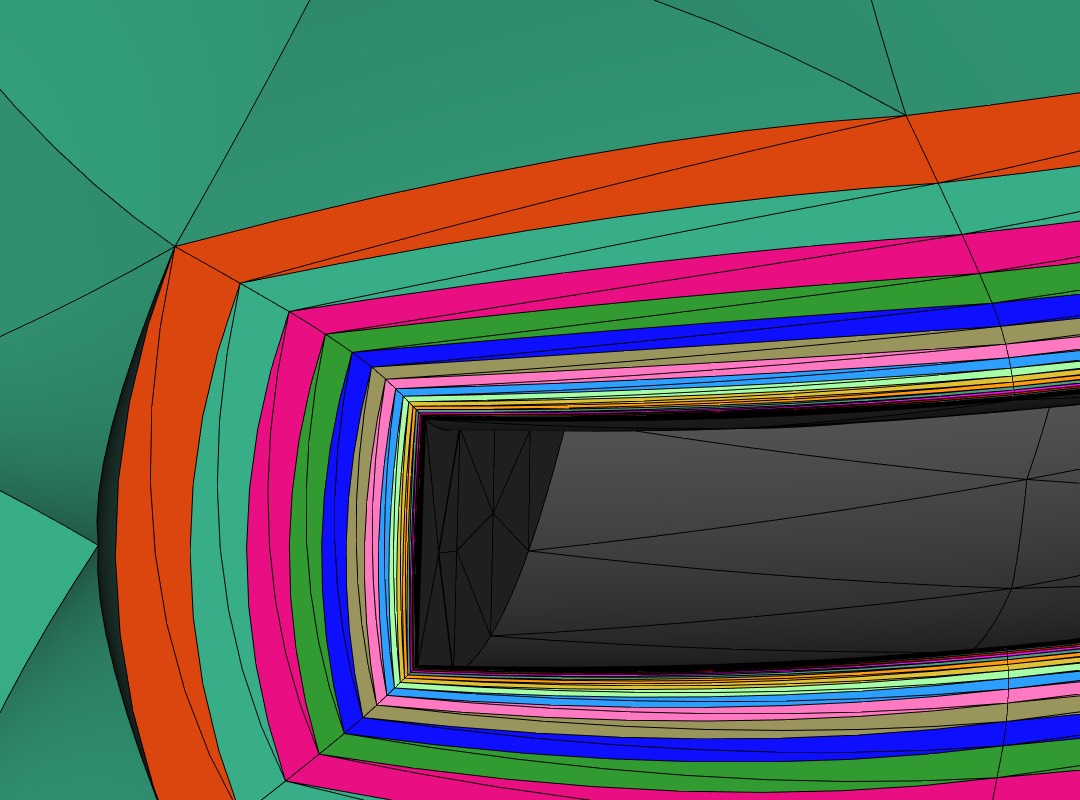
- Adaptation (extreme anisotropy, metric-aligned, metric-orthogonal).
- High-order (tetrahedra, hexahedra, boundary layer, adapted).
- Larges meshes (tetrahedra, hexahedra, adapted).
- Moving mesh methods for moving geometries.
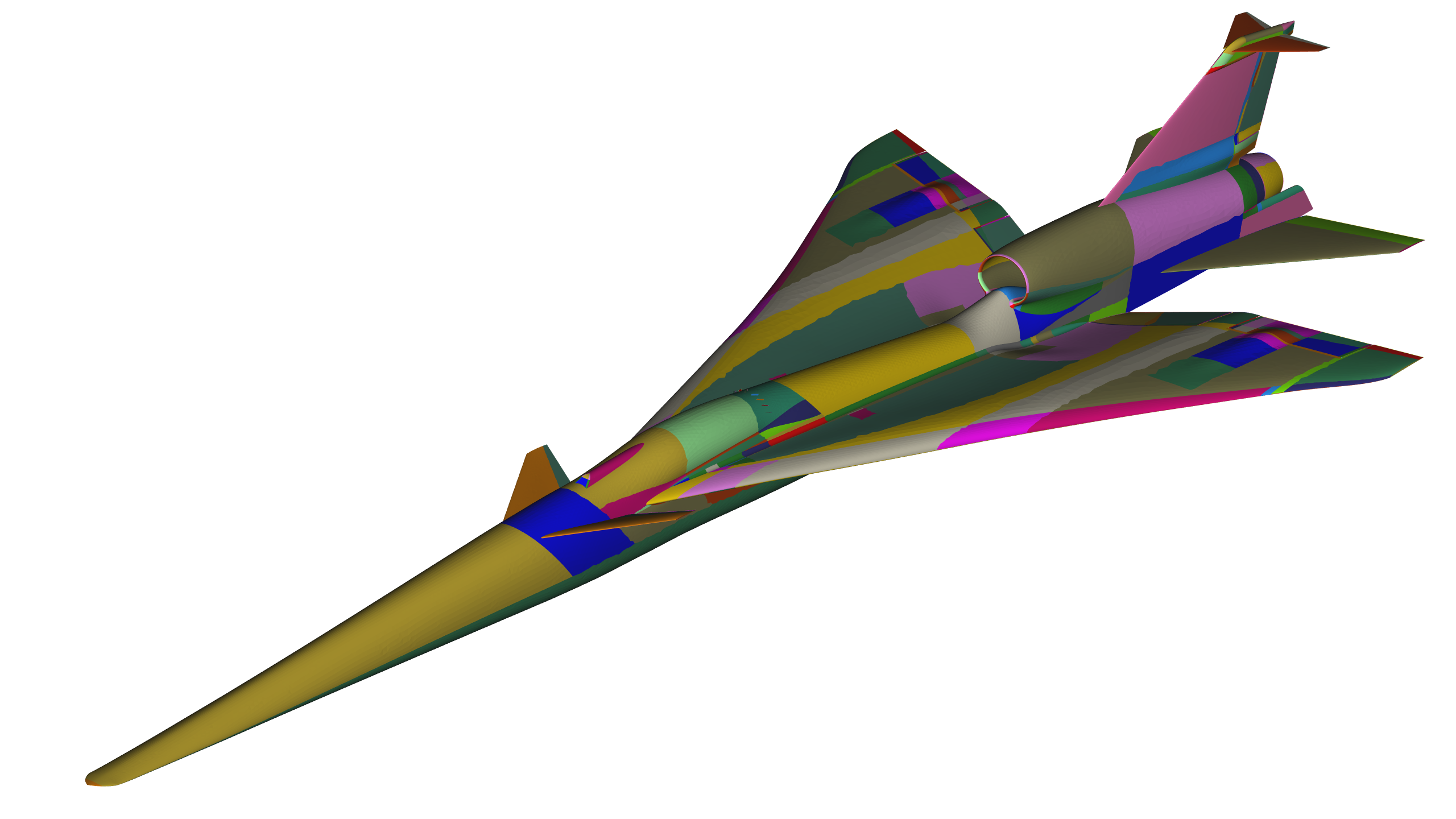
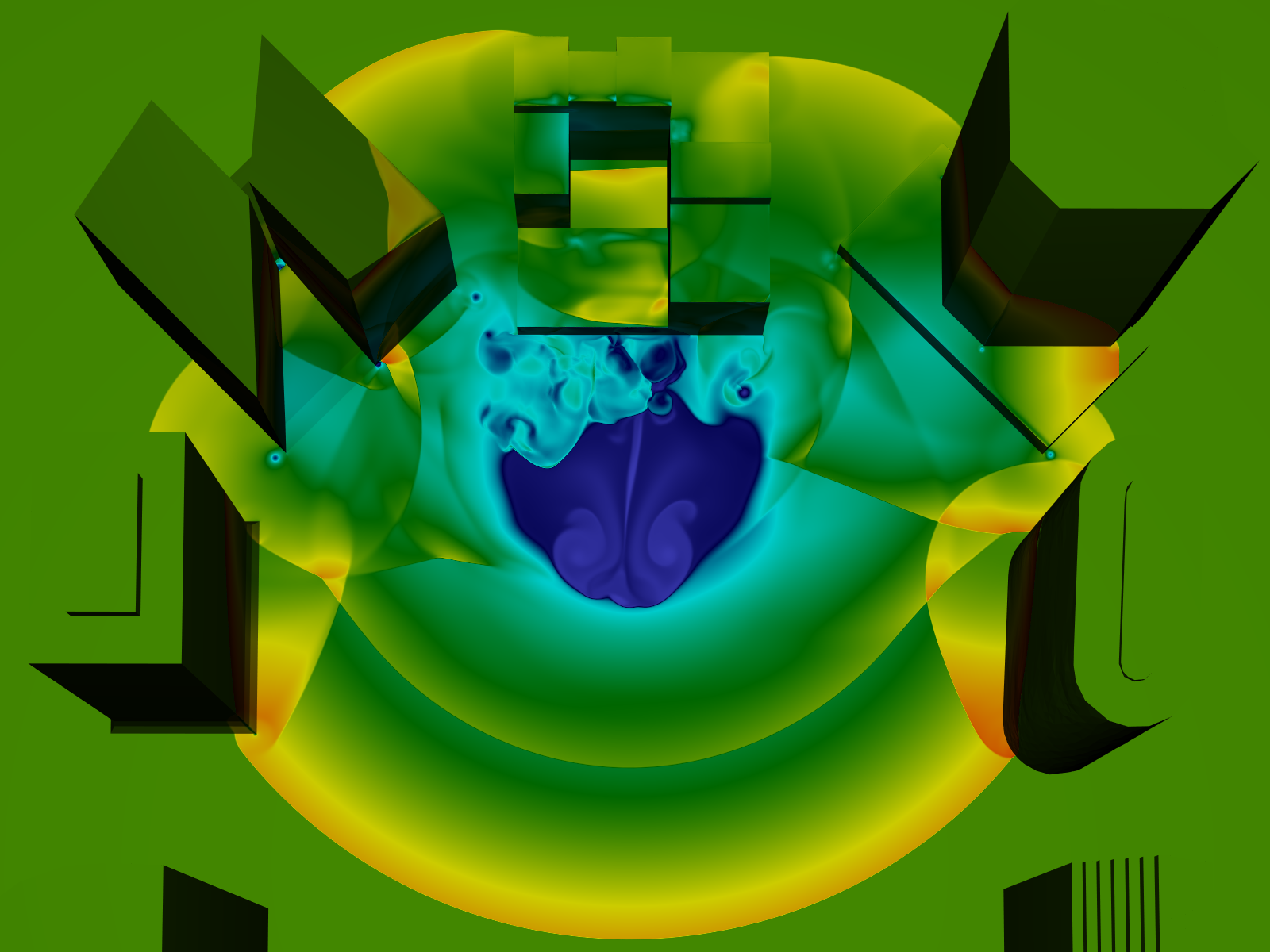
- Toward Certified Solutions to the Navier-Stokes Equations:
- Flow solver and adjoints (Finite Volumes, Finite Elements, Flux Reconstruction).
- Error estimates and correctors.
- Advanced Mesh and Solution Visualisation:
- Pixel exact rendering (High-Order mesh, High-Order solution).
- Pre-processing and post-processing.


International and industrial relations
- French academic relationships with Inria teams, ONERA, UPEC – Modélisation et Simulation Multi-Echelle, UPMC d’Alembert, l’X Laboratoire de Physique Théorique.
- International academic relationships with Mississipi State University, EPFL GR-PI, NASA Langley, MIT Aerospace Computational Design Laboratory, Stanford University Aerospace Design Lab.
- Industrial relationships with DISTENE, Lemma, Safran Tech, the Boeing Company, Total, Phimeca.
5 recent representative publications
-
Mesh adaptation allowed a major scientific discovery and made the cover page of Nature!
T. Amari, A. Canou, J.-J. Aly, F. Delyon, and F. Alauzet. Magnetic cage and rope as the key for solar eruptions. Nature, 554:211-215, 2018. [PDF] -
How to generate one billion of elements in 15 minutes
A. Loseille, F. Alauzet and V. Menier. Unique cavity-based operator and hierarchical domain partitioning for fast parallel generation of anisotropic meshes. Comput. Aided Des., 85 : 53-67, 2017. [PDF] -
First paper demonstrating that we can move complex geometries in highly anisotropic adaptive meshes
N. Barral, G. Olivier, and F. Alauzet. Time-accurate anisotropic mesh adaptation for three-dimensional time-dependent problems with body-fitted moving geometries. J. Comp. Phys., 331:157-187, 2017. [PDF] -
First paper demonstrating the superiority of mesh adaptation to solve turbulent flow on industrial applications
F. Alauzet and L. Frazza. Feature-based and goal-oriented anisotropic mesh adaptation for RANS applications in aeronautic and aerospace. J. Comp. Phys., 439, 110340:1-77, 2021. [PDF] -
First software enabling pixel-exact rendering of high-order solutions
R. Feuillet, M. Maunoury and A. Loseille. On pixel-exact rendering for high-order mesh and solution. J. Comp. Phys., 424, 109860:1-27, 2021. [PDF]
Team leader
Frédéric Alauzet, Projet GAMMA
INRIA Saclay – Ile de France
1 Rue Honoré d’Estienne d’Orves,
91120 Palaiseau
Frederic[point] Alauzet [at] inria [point] fr
